应用数学计算代写 数学计算代写 数据分析代写 数学作业代写
1052Fall 2021 应用数学计算代写 Read these instructions carefully!!! This project involves predicting what happens to a mortgage loans that have been purchased by FNMA during the Read these instr...
View detailsSearch the whole station
模块和表示论代写 1. (9 marks) Recall that an integral domain is a commutative ring (with unity) that has no zero divisors. (a) Prove that if R is an integral domain
(Due Friday, 18 March, 2022, 8pm)
Recall that an integral domain is a commutative ring (with unity) that has no zero divisors.
(a) Prove that if R is an integral domain, then the set of torsion elements in an R-module M (denoted Tor(M)) is a submodule of M.
(b) Give an example of a ring R and an R-module M such that Tor(M) is not a submodule.
(c) Show that if R has zero divisors, then every non-zero R-module has non-zero torsion elements.
Let R be a commutative ring and M an R-module.
(a) Show that HomR(R, M) can be given the structure of an R-module in a natural way. (Define this R-module structure explicitly, check that the structure you wrote down is well-defined, then check that it satisfies the axioms of an R-module.)
(b) Show that HomR(R, M) and M are isomorphic as R-modules.
(c) Show that EndR(R) and R are isomorphic as rings.
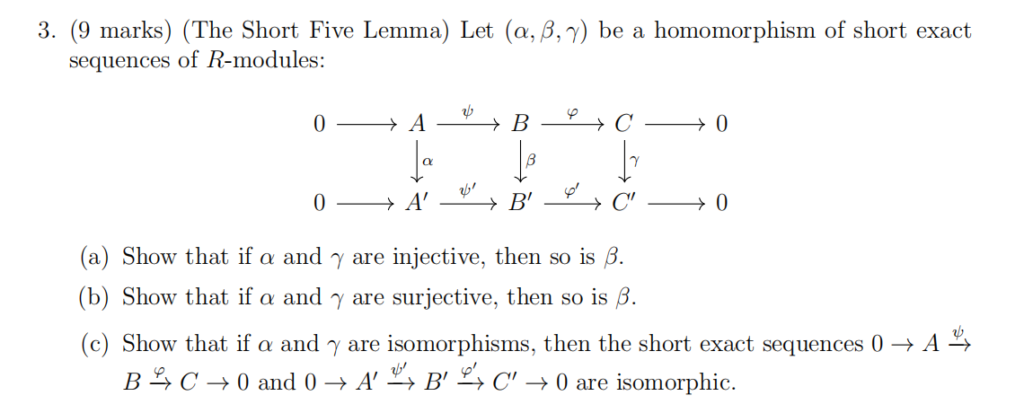
Prove that for every ring R, the following are equivalent.
(a) Every R-module is projective.
(b) Every R-module is injective.
Consider the C[x]-module M := C[x]/(x3 − x2 ), where (x3 − x2 ) is the ideal in C[x] generated by x3 − x2 .
(a) Show that M is finite length by constructing a composition series.
(b) Write down the composition factors of M.
(c) Is M Noetherian? Justify your answer.
(d) Is M Artinian? Justify your answer.

更多代写:java code辅导 gre?proctoru代考 英国物理Final exam代考 term paper怎麼寫 conclusion例子 文章网代写
合作平台:essay代写 论文代写 写手招聘 英国留学生代写
Fall 2021 应用数学计算代写 Read these instructions carefully!!! This project involves predicting what happens to a mortgage loans that have been purchased by FNMA during the Read these instr...
View details4. Probability on finite sample spaces. 数学概率作业代写 1. Are the following events A, B ⊂ Ωroulette independent? Find P(A|B) and P(B|A) in each case. a) A = Red, B = Even, 1. Are the fol...
View detailsMACM 201 - D100 AND D200 ASSIGNMENT #8 离散数学作业代写 Instructions Answer all questions on paper or a tablet using your own handwriting. Put your name, student ID number and page number at th...
View details数学代考的价格是多少?想找人帮忙代修和代考数学网课 国外数学网课代修 由于新冠肺炎疫情的影响,很多学校为了避免学生大规模的聚集,于是将课堂授课的模式改为了线上网课,由老师们录制教学视频,学生们在...
View details