数学统计作业代写 数学作业代写 统计作业代写 数学代写

Homework 3

数学统计作业代写 Instructions: Solve the problems in the spaces provided and save as a single PDF. Then upload the PDF to Canvas Assignments by the due date.

Instructions: Solve the problems in the spaces provided and save as a single PDF. Then upload the PDF to Canvas Assignments by the due date. The recommended procedure is to download and print the homework. Fill in your solutions. Then scan the document and upload to Canvas Assignments. If this is not feasible, you may solve the problems on your paper, scan your solutions, then upload to Canvas. Neatness and presentation are important. Late homework not accepted. Show all work. Total points: 30

1) 数学统计作业代写

Let Y₁, Y₂, . . . , Y_n be independent and identically distributed random variables from the Bernoulli distribution with parameter 0 < p < 1.

f(y|p) = p^y (1 − p)^1−y    y = 0, 1

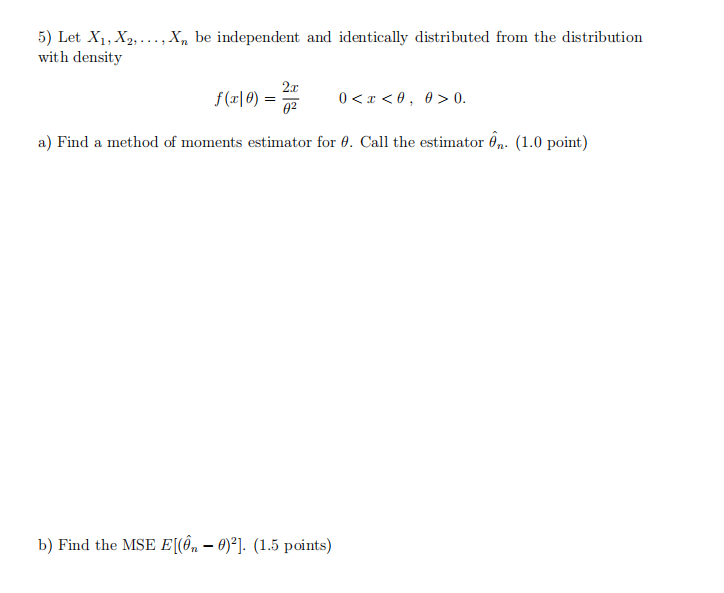
a) Find the method of moments estimator for p. (0.5 points)

b) Find the MLE (maximum likelihood estimator) for p. (1.5 points)

2)

Let Y₁, Y₂, . . . , Y_n be independent and identically distributed from the distribution with density

f(y| θ) = θ c^θ y^−(θ+1)             y > c.

where c > 0 is a constant and θ > 0. Find the MLE for θ. (1.5 points)

3) 数学统计作业代写

Let Y₁, Y₂ be an iid sample of size n = 2 from the pdf

f(y| θ) = 2yθ²             0 < y < 1/θ

Find the value c so that the statistic c(Y₁ + 2Y₂) is unbiased for 1/θ. (1.0 point)

4)

Let Y₁, Y₂, . . . Y_n be iid from the normal density N(µ, σ² ) and assume that σ² is known.

a) What is the Fisher information I₁(µ)? (1.5 points)

e) Let Y = max(X₁, . . . , X_n) = X_(n). Find the bias (2.0 points)

bY (θ) = E[Y ] − θ.

f) How can you correct Y to make it unbiased? (1.0 point)

c) Based on b) what is an approximate 95% confidence interval for θ? (0.5 points)

8) 数学统计作业代写

Let Y₁, Y₂, . . . , Y_n be independent and identically distributed from the geometric distribution with parameter 0 < θ < 1,

f(y| θ) = θ (1 − θ)^y−1   y = 1, 2, 3, . . .

c) Find an approximate 95% confidence interval for θ based on part b). (0.5 points)

10) Let Y ∼ N(θ, 1). 数学统计作业代写

a) Is this distribution a member of the exponential family? (1.5 points)

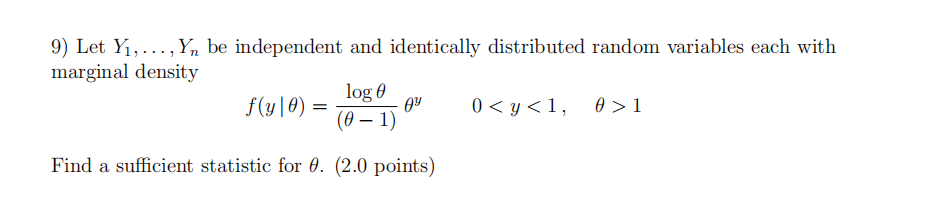
b) Now let Y₁, . . . , Y_n be iid N(θ, 1). Find a sufficient statistic for θ. (1.0 point)

11) Introduction to the Bootstrap.

#set the seed so we all get the same answers
set.seed(123)

#this command generates 1,000 multinomial random variables
simdat <- rmultinom(1000,size=1029,prob=c(0.331,0.489,0.180))

#this is a function to calculate the MLE
thfun <- function(y) {
  (2*y[3] + y[2])/(2*1029) }

#this command applies the function to the columns of the simulated data
theta <- apply(simdat,2,thfun)

#makes a histogram, calculates mean and standard error
hist(theta,freq=F,col="lightblue"); mean(theta); sd(theta)

更多代写：CS加拿大网课代修  雅思线上考试  英国微积分网课代上  研究论文内容代写  北美留学生essay代写  Sci代写代发

合作平台：essay代写 论文代写 写手招聘 英国留学生代写