生产和运营分析代写 IEOR 3402代写 运筹学代写 考试助攻

IEOR 3402: Midterm Examination 1 (Spring 2021)

生产和运营分析代写 You are allowed to use the textbook. No other aid (e.g. calculator) allowed. Duration: 75 minutes. 8:40am EST till 9:55am EST.

• You are allowed to use the textbook. No other aid (e.g. calculator) allowed.
• Duration: 75 minutes. 8:40am EST till 9:55am EST.

Copy the following Honor Pledge on the fifirst page of your submission and sign it. Without the Honor Pledge your exam will not be graded.

1.(13 points)

Consider the following data for demand at the NYC Babysitting Company.

Year	Actual Demand
1	200
2	250

Suppose we make yearly demand forecasts using Holt method. Assume that both smoothing constants are equal to 0.1. Suppose S₂ = 190 and G₂ = 10.

(a) (5 points) Suppose that the actual demand in Year 3 is 175. Compute S₃ and G₃. Simplify your solution.

(b) (8 points) Compare the demand forecast for Year 4 made at the end of Year 2, and the

demand forecast for Year 4 made at the end of Year 3. If the actual demand in Year 4 is 215, which forecast was more accurate? Simplify your solutions.

2.(22 points) 生产和运营分析代写

We consider an aggregate planning model for multiple products in which there is an upper and a lower bound for the demand in each period and we can sell any number within these bounds. The objective is to maximize the net profit minus inventory carrying cost subject to upper and lower bounds on sales and capacity constraints. We use the following notation:

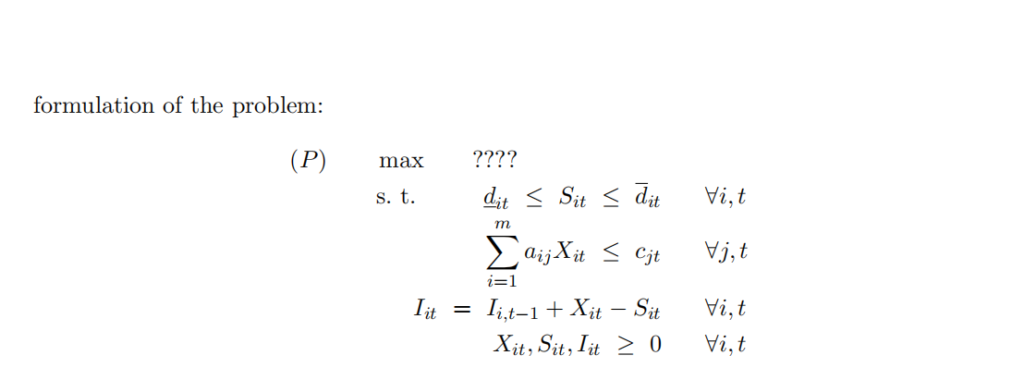
If you use new variables in any section please define them clearly.

(a) (5 points) Write the objective function of the linear programming formulation (P).

(b) (5 points) In (P), we forced inventory to remain positive at all times. Implicitly, we were assuming that demands had to be met from inventory or lost; no backlogging of unmet demand was allowed. However, in many situations, demand is not lost when it is not met on time; customers expect to receive their orders even when they are late. Let π_i represent the penalty to carry one unit of product i on backorder for one period of time. Modify (P) to give an appropriate linear programming formulation. 生产和运营分析代写

(c) (5 points) In (P), we assumed that each workstation is available for a fixed amount of time in each period. In many systems, there is the possibility of increasing the time via the use of overtime. Let δ_j be the per-unit cost of overtime at workstation j. Modify (P) to give an appropriate linear programming formulation. Ignore changes made in (b) for this section.

(d) (7 points) Suppose now we have decided to supplement the capacity by subcontracting parts of the production of certain products. Let k_it be the per unit premium paid for subcontracting product i in period t (i.e., cost above variable cost of making it in-house, c_i). Let v_i be the minimum amount of product i that must be purchased over the time horizon (e.g., specified as part of the long-term contract with the supplier). Modify (P) to give a linear programming formulation for this scenario. Ignore changes made in (b) and (c) for this section.

3. (25 points) 生产和运营分析代写

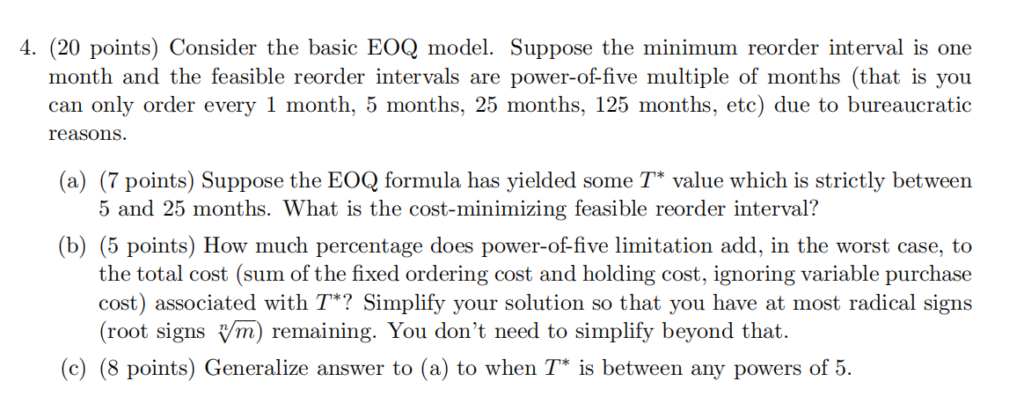
Consider the basic (single-product) EOQ model with parameters λ, K, c and I. The objective is to minimize the sum of the time-average holding and fixed order costs.

(a) (4 points) Write the objective function with respect to the cycle time T.

(b) (8 points) Consider the following multi-product system. Let n be the number of products, indexed by i = 1, . . . , n. For each product i, the demand rate is λ_i and the purchase cost is ci per unit. The fixed cost of K is incurred whenever an order is placed, regardless of the number of products ordered. Let I be the interest rate. Does it make sense that each time an order is placed, every product is ordered? Explain. Be as rigorous as possible.

(c) (13 points) Not a particulary easy section! Make sure you solve easier problems first! Consider the problem in part (b). Suppose that each time an order is placed, every product is ordered. What is the optimal ordering policy? Justify your answer. You can answer this part without answering part (b).

5. (20 points) 生产和运营分析代写

Consider EOQ model with planned backorders. In class, we discussed the setting where each unit of backlogged inventory incured a penalty per unit of time ($b per unit per-time period.) Now consider an alternative setting, where each unit of backlogged inventory incurs a one time penalty cost of size $d regardless of the length of the backlog. There is no other change. You still have to satisfy the demand fully in each cycle.

(a) (8 points) Write the total cost function per cycle. Ignore the variable purchase cost.

(b) (4 points) Write the cost function per unit of time. Ignore the variable purchase cost.

(c) (8 points) Consider a hypothetical scenario where for operational reasons you have to order Q units where Q is given to you and you cannot change it. What is the optimal re-order point based on the cost function you derived in (b).

更多代写：Cs网课quiz  托福家考保分  英国网课assignment代写  网课essay代写论文  推荐格式代写 我们的未来气候代写

合作平台：essay代写 论文代写 写手招聘 英国留学生代写