宏观经济原理试题代写 EC210代写 Macroeconomic代写
EC210 Macroeconomic Principles
宏观经济原理试题代写 Instructions This exam contains two sections. Answer all questions. The two short questions in Section A carry 20 marks each, and the one long
Instructions
This exam contains two sections. Answer all questions.
The two short questions in Section A carry 20 marks each, and the one long question in Section B carries 60 marks. Do not spend a disproportionate amount of time on any one question.
If at any point in this exam you feel that anything is unclear, please make additional assumptions that you feel are necessary and state them clearly.
Time Allowed Reading Time: 15 minutes
Writing Time: 1 hour
You are supplied with: No additional materials
You may also use: No additional materials
Calculators: Calculators are not allowed in this exam
Section A 宏观经济原理试题代写
(Answer both questions below.)
Question 1
The following observations are made of prices and quantities of goods purchased by consumers in an economy over two years.
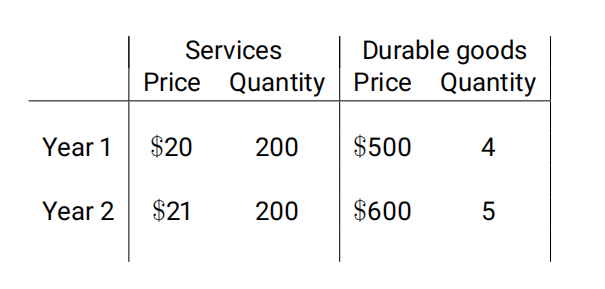
Assuming the consumer price index (CPI) is based on the basket of goods from year 1, calculate the CPI inflation rate between years 1 and 2. Is the increase in the cost of buying the year-2 basket of goods between the two years greater or smaller than CPI inflation? Does this example illustrate the substitution bias of the CPI? Briefly explain your answer. [20 marks]
Question 2 宏观经济原理试题代写
Suppose that an economy has the following average annual growth rates of its real GDP Y , capital stock K, and population N over a decade.
| Variable | GDP (Y ) | Capital stock (K) | Population (N) |
| Growth rate | 4% | 4% | 1% |
Assuming the economy’s production function is Y = zK1/3N2/3, where z is total factor productivity (TFP), perform a growth accounting exercise to break down the 4% growth of GDP into parts attributable to growth in the capital stock, population, and TFP.
Explain why this example is consistent with the balanced growth path of the Solow model with a constant rate of labour-augmenting technological progress, that is, where A grows at a constant rate in the production function Y = K1/3(AN)2/3. Supposing there were no further labour-augmenting technological progress in subsequent decades, does subtracting the contribution of TFP growth in the growth-accounting exercise from the earlier 4% growth rate give the model’s prediction for what will happen to the GDP growth rate in the long run? Briefly explain. [20 marks]
Section B 宏观经济原理试题代写
(Answer the one question below.)
Question 3
Consider an economy where aggregate output Y is produced using capital K and labour N according to the Cobb-Douglas production function Y = √K √AN, where A represents technology that augments labour N so that effective labour input is AN. The population N is constant in this question (N’ = N, so the rate of population growth n is zero), and assume that N = 1 as a simplification, which means Y and K are the same as output per worker y = Y/N and capital per worker k = K/N respectively.
The change in the capital stock over time is K’−K = I −0.08K, where I is investment and d = 0.08 is the depreciation rate (8%). Investment is equal to saving sY , where s is the saving rate. Initially, assume that A is constant over time. [60 marks]
(a)
Write down an equation for the capital stock K in a steady state where K0 = K, and use a diagram plotting the ‘saving line’ sY and ‘depreciation line’ 0.08K against K to show that such a steady state exists. [8 marks]
The return on investment after depreciation is r = ∂Y/∂K − 0.08, the marginal product of capital ∂Y/∂K minus the depreciation rate d = 0.08. This is the return received by savers.
(b) 宏观经济原理试题代写
Show that the marginal product of capital is Y/(2K), so r = Y/(2K) − 0.08. [4 marks]
Suppose households in the economy are very farsighted and increase their saving until the return on investment after depreciation is zero (r = 0).
(c)
Using your answers to (a) and (b), what saving rate s results from this behaviour? [8 marks]
(d)
Briefly explain why consumption C = (1−s)Y is at its highest possible long-run level when the saving rate is the one found in part (c). No mathematical derivation is required. [8 marks]
Now assume there is ‘learning by doing’. Specifically, there is a positive spillover from capital accumulation to knowledge whereby A = (1+0.12√K)2 . This means knowledge A increases with the capital stock K, but the two are not exactly proportional here.
(e)
Show the learning-by-doing effect implies output is given by Y = √K + 0.12K. [4 marks]
(f) 宏观经济原理试题代写
For the saving rate s found in part (c), use a diagram with saving and depreciation lines sY and 0.08K to explain why the learning-by-doing effect does not generate endogenous long-run growth. [Hint: explain why the saving line is not always above the depreciation line.] [8 marks]
(g)
Using the diagram from part (f), show that there are higher saving rates s that would lead to positive long-run growth. How large does the saving rate need to be for this? [8 marks]
Knowledge is a public good and the level of A is taken as given by those making saving and investment decisions. Thus, the equation for the return r received on investments is the same as in part (b).
(h)
At the steady state for K from part (f), show that r = 0. [4 marks]
(i)
If the objective is to raise consumption C in the long run, explain why there is a case for the government to give subsidies to invest so that investment occurs even when r < 0. To maintain positive long-run growth, how far below zero will r need to fall? [8 marks]

更多代写:CS php代码代写 proctorio作弊 英国PHIL哲学代写 Philosophy哲学论文代 paper统计学代写 留学英文简历代写
合作平台:essay代写 论文代写 写手招聘 英国留学生代写
 作业代写
作业代写