代写数学作业 MATH 7241代写 数学作业代写 数学课业代写

MATH 7241

代写数学作业 Problem Set #3 Reading: relevant background material for these problems can be found in the class notes, and in Rosenthal Chapter 3.

Problem Set #3 代写数学作业

Reading: relevant background material for these problems can be found in the class notes, and in Rosenthal Chapter 3.

Exercise 1

Let X₁, X₂,… be a sequence of random variables (not necessarily independent), and suppose that E[X_n] = 0 and E[(X_n)²] = 1 for all n ≥ 1. Prove that

P(X_n ≥ n i.o.)=0

where i.o. means ‘infinitely often’. [Hint: define the event A_n = {X_n ≥ n} and use the first Borel-Cantelli Lemma along with Markov’s inequality]

Exercise 2

Let X₁, X₂,… be independent random variables and suppose that X_n is uniform on the set {1, 2,…,n} for each n ≥ 1. Compute P(X_n =5 i.o.). [Hint: use the Borel-Cantelli Lemma]

Problem Set #4 代写数学作业

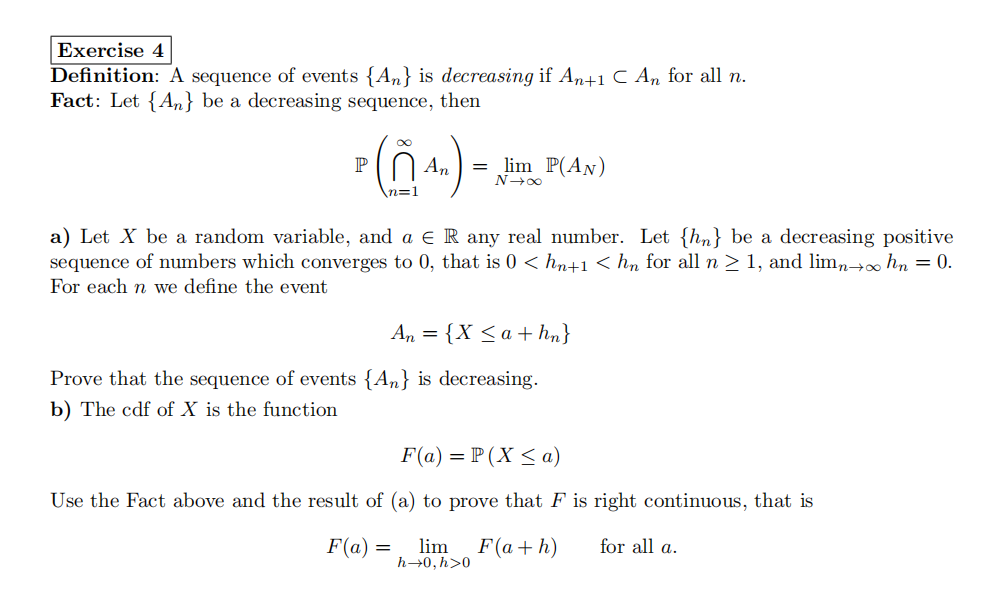
Reading: relevant background material for these problems can be found on Canvas ‘Notes 4: Finite Markov Chains’. Also Grinstead and Snell Chapter 11.

Exercise 1 ‘Finite Markov Chains – Problems’, Exercise 4.

Hint: draw a graph with 6 nodes to represent the states of the chain, and draw a directed edge between each pair of nodes (i, j) for which the transition matrix entry p_ij is positive. You can identify the set of transient states as the group of nodes from which edges exit, but into which there are no entering edges. Once you find the transient states, the remaining states are all irreducible, and break up into subsets which intercommunicate.

Exercise 2 ‘Finite Markov Chains – Problems’, Exercises 5 a), 5 b).

Exercise 3 ‘Finite Markov Chains – Problems’, Exercise 2.

Hint: to represent this by a Markov chain you must use the result of two successive trials as your state. So there are four states: SS, SF, F S, F F where S is success and F is failure, and SF means success on trial n and failure on trial n + 1. Then if your current state is SF, your next state must be either F S or F F.

*Exercise 4 ‘Finite Markov Chains – Problems’ file: Exercise 3

Exercise 4 Grinstead & Snell, p. 442: #2.

Note: see pages 442-443 from Grinstead and Snell on Canvas. The text is available online (free!) at

http://www.dartmouth.edu/⇠chance/

Click on the link “A GNU book”.

更多代写：d5.js代写  线上考试作弊被发现怎么办  英国fin代考价格  会计论文代写  如何写summary  stata代写网站

合作平台：essay代写 论文代写 写手招聘 英国留学生代写